Here are the titles and abstracts of the seminars which were held in 2007:
2007-12-04 Seminar
Marcin BorkowskiOn some Krasnoselskii type theorems
We will prove two fixed point theorems of Krasnoselskii type.
2007-11-27 Seminar
Danuta Borowska and Jerzy GrzybowskiIntersection property in the class of all closed bounded and convex sets.
(Continuation of the 2007-11-13 Seminar)
2007-11-20 Seminar
P. Kawęcki, B. Kraska, K. Majcherek, M. Zoła (lecturer: B. Kraska)
(John Paul II Catholic University of Lublin)
Guarding a line segment
A line segment on the plane, equipped with norm 5c7c203d205cmax205c7b7cx_7b17d7c2c207cx_7b27d7c5c7d202424.png) , is guarded by the defender D. The invader I wants to reach the line segment but he has to keep the distance from the defender no less than a given constant
, is guarded by the defender D. The invader I wants to reach the line segment but he has to keep the distance from the defender no less than a given constant 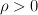 . The defender and the invader can move on the whole plane with maximal speed of
. The defender and the invader can move on the whole plane with maximal speed of 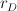 and
and 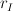 respectively, where
respectively, where 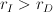 . No further kinematic or dynamic constrains are imposed on the defender and the invader motions. The maximal length of the line segment which can be guarded by the defender is established in this paper.
. No further kinematic or dynamic constrains are imposed on the defender and the invader motions. The maximal length of the line segment which can be guarded by the defender is established in this paper.
2007-11-13 Seminar
Danuta Borowska and Jerzy GrzybowskiIntersection property in the class of all closed bounded and convex sets.
We will discuss the following property of compact convex sets: nonempty intersection of an arbitrary class of translations of a set is a summand (in the Minkowski sense) of the set. We will show that some sets different to polyhedron and ellipsoid, such as wedges, dull wedges and some parts of the Euclidean ball also own the property in question. Moreover, we will prove that the family of all three-dimensional strongly monotypical polyhedrons coincide with the family of all three-dimensional polyhedral sets having the above property.
2007-11-06 Seminar
Marcin BorkowskiHyperconvex hull revisited
In the talk we will consider hyperconvex spaces with the property that each subset has a unique hyperconvex hull. A necessary and sufficient condition for a space to possess this property will be given.
2007-10-23 Seminar
Hubert PrzybycieńOn the space of  -convex functions and its dual
-convex functions and its dual
The talk is based on the paper "On the space of  -convex functions and its dual" by Urban Cegrell.
-convex functions and its dual" by Urban Cegrell.
2007-10-16 Seminar
Marcin BorkowskiHyperconvexity and convexity
We will discuss the relation between hyperconvexity and convexity in Banach spaces. We will state the conditions a Banach space must satisfy so that convexity imply hyperconvexity and conversely.
2007-10-09 Seminar
Ryszard UrbańskiOn minima of families of sublinear functions
In the talk a necessary and sufficient condition for a minimum of finitely many elements of the Minkowski-Rådström-Hörmander space to belong to the cone of nonempty, bounded, closed and convex sets will be given. This result will be applied to show a condition for a minimum of a finite family of support functions to be sublinear.
2007-05-29 Seminar
Ryszard Urbański and Jerzy GrzybowskiConvex and Fractal Geometry conference in Będlewo -- report
2007-05-15 Seminar
Jerzy GrzybowskiOn crystal growth
We will present a model of crystal growth and a sufficient and necessary condition for vanishing of facets. We will also compute the derivative and propose a formula for the shape of the crystal at any given moment.
2007-05-08 Seminar
Marcin BorkowskiDarbo type theorems for multifunctions
We will present some Darbo-type theorems for multivalued operators in hyperconvex metric spaces.
(Continuation of the 2007-04-17 Seminar)
2007-04-25 Seminar
This lecture will be held on Wednesday at 12.30
prof. dr hab. Andrzej Fryszkowski (Faculty of Mathematics and Information Science at the Warsaw University of Technology)
Filippov lemma for differential inclusions of higher orders
2007-04-17 Seminar
Marcin BorkowskiDarbo type theorems for multifunctions
We will present some Darbo-type theorems for multivalued operators in hyperconvex metric spaces.
2007-03-28 Seminar
This lecture will be held on Wednesday at 12.30 in room B3-39
prof. dr hab. Tadeusz Rzeżuchowski (The Faculty of Mathematics and Information Science at the Warsaw University of Technology)
On the equivalent differential inclusions and minimal assumptions about convexity of the right side
2007-03-20 Seminar
Hubert PrzybycieńOn the completeness of the Minkowski-Rådström-Hörmander space
We will consider the problem of completeness of the Minkowski-Rådström-Hörmander space  in the case of
in the case of 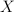 being a metrizable topological vector space.
being a metrizable topological vector space.
2007-03-13 Seminar
Ryszard Urbański and Jerzy GrzybowskiExposed points of the unit ball in the Minkowski-Rådström-Hörmander Space
(Continuation of the 2007-03-06 Seminar)
2007-03-06 Seminar
Ryszard Urbański and Jerzy GrzybowskiExtreme points of the unit ball in the Minkowski-Rådström-Hörmander Space
(Continuation of the 2007-02-27 Seminar)
2007-02-27 Seminar
Ryszard Urbański and Jerzy GrzybowskiExtreme points of the unit ball in the Minkowski-Rådström-Hörmander Space
During the lecture we will prove that the unit ball in the Minkowski-Rådström-Hörmander Space  over any normed vector space
over any normed vector space 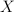 ,
, 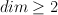 , has exactly two extreme points. This result is an effect of the characterization of extreme points of any symmetric interval in the Minkowski-Rådström-Hörmander vector lattice
, has exactly two extreme points. This result is an effect of the characterization of extreme points of any symmetric interval in the Minkowski-Rådström-Hörmander vector lattice  over any Hausdorff topological vector space
over any Hausdorff topological vector space 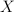 .
.
2007-01-23 Seminar
Paweł SuplickiOn functions represented as a difference of two convex functions
(Continuation of the 2007-01-16 Seminar)
2007-01-16 Seminar
Paweł SuplickiOn functions represented as a difference of two convex functions