Ryszard Urbański and Jerzy GrzybowskiExtreme points of the unit ball in the Minkowski-Rådström-Hörmander Space
During the lecture we will prove that the unit ball in the Minkowski-Rådström-Hörmander Space  over any normed vector space
over any normed vector space 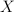 ,
, 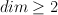 , has exactly two extreme points. This result is an effect of the characterization of extreme points of any symmetric interval in the Minkowski-Rådström-Hörmander vector lattice
, has exactly two extreme points. This result is an effect of the characterization of extreme points of any symmetric interval in the Minkowski-Rådström-Hörmander vector lattice  over any Hausdorff topological vector space
over any Hausdorff topological vector space 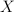 .
.