Here are the titles and abstracts of the seminars which were held in 2008:

2008-12-16 Seminar

mgr Paweł Suplicki
Convex Programming

In the talk we will discuss convex programming. Furthermore, we will present symplex algorithm introduced by G.B. Dantzig.

2008-12-09 Seminar

prof. dr hab. Tadeusz Rzeżuchowski (The Faculty of Mathematics and Information Science at the Warsaw University of Technology)

Demyanov metric in the family of convex bounded sets

The seminar will be held at 9.00 in A1-33.

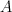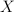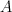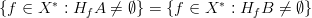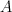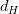Let $f$ be a linear and continuous functional $f$ and $A$ a convex bounded subset of a linear space $X$ . We call the set $\{x\in A:f(x)=\sup_{y\in A}f(y)\}$ the face of $A$ supported by the function $f$ and denote it by $H_fA$ . If $\{f\in X^*:H_fA\ne\emptyset\}=\{f\in X^*:H_fB\ne\emptyset\}$ , we define the Demyanov distance between the sets $A$ and $B$ as $\sup_f d_H(H_fA,H_fB)$ , where $d_H$ is the usual Hausdorff metric.

2008-12-02 Seminar

Paweł Suplicki
Linear Programming

(Continuation of the 2008-11-25 Seminar)

2008-11-25 Seminar

Paweł Suplicki
Linear Programming

In the talk we will discuss linear and convex programming. Moreover, we will give some examples as well as applications.

2008-11-18 Seminar

mgr Danuta Borowska
On some representations of linear functionals

(Continuation of the 2008-10-28 Seminar)

2008-11-04 Seminar

Ryszard Urbański i Jerzy Grzybowski
Shadowing set and finite exhausters

(Continuation of the 2008-10-07 Seminar and the 2008-10-21 Seminar)

2008-10-28 Seminar

mgr Danuta Borowska
On some representations of linear functionals

2008-10-21 Seminar

Ryszard Urbański and Jerzy Grzybowski
Shadowing set and finite exhausters

(Continuation of the 2008-10-07 Seminar)

2008-10-07 Seminar

Ryszard Urbański and Jerzy Grzybowski
Shadowing set and finite exhausters

We will introduce the notation of a shadowing set, which is a generalization of the notion of separating set to the family of more than two sets. We will prove some theorems dealing with the shadowing set. Furthermore, we will give the criterion for upper exhauster to be an exhauster of sublinear function as well as the criterion for minimality of finite upper exhauster. All the results will be illustrated by examples.

2008-06-10 Seminar

Mahide Küçük (Anadolu University, Eskişehi, Turcja)

Optimalization over sets defined by inequality constraints

(The lecture will be held at 12.00 in room A1-23)

In the talk, firstly Non Linear Programming problems (NLP) will be presented in 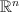$\mathbb{R}^{n}$ . Then first order necessary condition (Karush-Kuhn-Tucker Theorem), second order necessary condition and one sufficient condition under inequality constraints for NLP problems will be given. This will be followed by examples about the subject. Finally, two sufficient condition for Constrained Qualification (CQ) of NLP problems will be given.

2008-05-13 Seminar

Since prof. Urbański and dr Grzybowski take part in a conference, the seminar on May 13 is cancelled.

2008-05-06 Seminar

Danuta Borowska

Properties of the Minkowski subtraction of convex sets

(Continuation of the 2008-04-29 Seminar)

2008-04-29 Seminar

Danuta Borowska

Properties of the Minkowski subtraction of convex sets

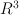In the talk we will define the Minkowski sum and subtraction in a semigroup. Furhtermore, we will prove some properties of the Minkowski subtraction, summands and the Sallee's sets in the semigropu $K(X)$ . We will also discuss the full classification of strongly monotypic polyhedrons in $R^{3}$ and the relationship between the strongly monotypic polyhedrons and the Sallee's sets.

2008-04-22 Seminar

Hubert Przybycień

On a topological direct sum decomposition of a topological group $\tilde{S}$

(Continuation of the 2008-04-15 Seminar)

2008-04-15 Seminar

Hubert Przybycień

On a topological direct sum decomposition of a topological group $\tilde{S}$

(Continuation of the 2008-04-08 Seminar)

2008-04-08 Seminar

Hubert Przybycień

On a topological direct sum decomposition of a topological group $\tilde{S}$

We will prove a topological direct sum decomposition theorem of a topological group $(\tilde{S},+)$ into its two subgroups: symmetric and asymetric one, under some assumptions about uniformly continuity of + and so-called symetry operator. Furthermore, we will give some examples.

2008-04-01 Seminar

Hubert Przybycień

On a direct sum decomposition of a group 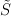$\tilde{S}$

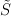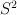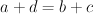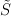Given a commutative semigroup with the neutral element and the cancellation law $(S,+)$ , denote by $\tilde{S}$ the set $S^{2}$ with the equivalence relation $R$ such that $(a,b)R(c,d)$ if and only if $a+d=b+c$ . We will show that $(\tilde{S},+)$ is a group and under some assumptions it is possible to decompose $\tilde{S}$ into a direct sum of two subgroups: symmetric and asymmetric one.

2008-03-18 Seminar

Danuta Borowska

On decomposition of closed, convex set theorems.

(Continuation of the 2008-03-11 Seminar)

2008-03-11 Seminar

Danuta Borowska

On decomposition of closed, convex set theorems.

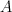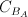We will present Klee-Minkowski-Hirsch theorem, which states that every closed, convex set $A$ can be decomposed into linearity space $L_{A}$ , asymptotic cone $C_{B_A}$ and convex hull of extremal points of the intersection of 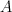$A$ and the orthogonal complement of the linearity space. We will also prove that the above theorem is a generalization of well-known results.

2008-03-04 Seminar

Piotr Kasprzak

Orthogonality in Birkhoff sense and semi-inner products in Banach spaces

(Continuation of the 2008-02-26 Seminar)

2008-02-26 Seminar

Piotr Kasprzak

Orthogonality in Birkhoff sense and semi-inner products in Banach spaces

We will introduce the notion of the orthogonality in Birkhoff sense and semi-inner products in Banach spaces. Furthermore, we will present some of their properties and give some examples.

2008-02-19 Seminar

Marcin Borkowski will continue his 22th January talk On orthogonality in normed spaces.

2008-01-22 Seminar

Marcin Borkowski
On orthogonality in normed spaces

We will discuss two ways of defining orthogonality in spaces without an inner product. The talk will be based on the classical paper by R. C. James.

2008-01-10 Seminar

prof. dr hab. Andrzej Cegielski (Faculty of Mathematics, Computer Science and Econometrics, University of Zielona Góra)

Regular nonexpansive mappings and their applications

2008-01-08 Seminar

Marcin Borkowski
On some Krasnoselskii type theorems

(Continuation of the 2007-12-04 Seminar)