Last edit
Changed:
< **Formal Power Series and the Boundary Convergence of Power Series**
< The behaviour of power series on boundary of convergence domain has been an interesting topic since power series was introduced. For example,
< $$ f(x) = \sum_{n=1}^{\infty}\dfrac{(-1)^n}{n}x^n$$
< converges on $$(-1,1]$$ but diverges at $$x = -1$$, and $$ f^{\prime}(x) $$ diverges at both $$x = 1$$ and $$x = -1$$. The composition of formal power series has been an important part of the //formal power series theory//. We introduce some relationship between these two subjects and provide a condition for convergence of a power series at every point in its interval of convergence, including endpoints or boundary points.
to
> **Composition and Generalized Composition of Formal Power Series**
> Given two formal power series
> $$f(z) = \sum_{n=1}^{\infty} a_n z^n$$ and $$g(z)=\sum_{n=0}^{\infty} b_n z^n$$
> the composition $$g \circ f$$ has been a very interesting topic for many years, where $$f$$ is called the nonunit. If $$ f(z) =\sum_{n=0}^{\infty} a_n z^n$$ with $$a_0 \neq 0$$, such a composition $$g \circ f$$ is called the generalized composition if it exists. We introduce the history of these two compositions and some results of them. Both of them are applied widely although the generalized composition is much younger than the regular one.
Xiao-Xiong Gan
(Mathematics Department, Morgan State University, Baltimore, USA)
Composition and Generalized Composition of Formal Power Series
Given two formal power series
203d205csum_7bn3d17d5e7b5cinfty7d20a_n20z5en202424.png) and
and 3d5csum_7bn3d07d5e7b5cinfty7d20b_n20z5en202424.png)
the composition  has been a very interesting topic for many years, where
has been a very interesting topic for many years, where  is called the nonunit. If
is called the nonunit. If 203d5csum_7bn3d07d5e7b5cinfty7d20a_n20z5en202424.png) with
with 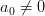 , such a composition
, such a composition  is called the generalized composition if it exists. We introduce the history of these two compositions and some results of them. Both of them are applied widely although the generalized composition is much younger than the regular one.
is called the generalized composition if it exists. We introduce the history of these two compositions and some results of them. Both of them are applied widely although the generalized composition is much younger than the regular one.
The seminar will take place at 9:00.