Marcin Borkowski
Fixed point theorems with a boundary condition
In 1998, Espínola and López proved a certain fixed point theorem for a mapping transforming an admissible subset 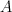 of a hyperconvex metric space into the whole space, assuming that this mapping sends the boundary of
of a hyperconvex metric space into the whole space, assuming that this mapping sends the boundary of 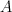 into
into 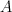 . We will show how to simplify their proof considerably, at the same time relaxing its assumptions. In particular, this will enable proving a new fixed point theorem, generalizing a classical result of Baillon from 1988.
. We will show how to simplify their proof considerably, at the same time relaxing its assumptions. In particular, this will enable proving a new fixed point theorem, generalizing a classical result of Baillon from 1988.