Dr Jacek Gulgowski
(Institute of Mathematics, Gdańsk University)
On the continuity of superposition operator in the spaces of functions of bounded variation
It is a well-know fact that the superposition of a function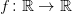 
satisfying a local Lipschitz condition with a function 202424.png)
of bounded variation leads to the function (t)202424.png)
which is also of bounded variation. During the seminar we are going to present a few results concerning the continuity of such a superposition operator ![$$ F \colon BV[0,1]\to BV[0,1] $$](http://octd.wmi.amu.edu.pl/latex/242420F205ccolon20BV5b02c15d5cto20BV5b02c15d202424.png)
. Furthermore, we are going to discuss several generalizations of the concept of bounded variation in the sense of Jordan and investigate the issue of continuity of the superposition operator ![$$ F \colon BV[0,1]\to E $$](http://octd.wmi.amu.edu.pl/latex/242420F205ccolon20BV5b02c15d5cto20E202424.png)
, where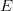 
denotes the space of functions of bounded variation in the generalized sense.