Last edit
Changed:
< During this lecture we will show that a Levitan almost periodic (briefly: LAP) solution to the equation $$y(x)=\lambda y(x)+f(x)$$ usually can be expressed by means of convolution. We will show that in this way we can get an unbounded LAP solution. We will give an example showing that the convolution in not always a good tool to look for LAP solutions.
to
> During this lecture we will show that a Levitan almost periodic (briefly: LAP) solution to the equation $$y$$'$$(x)=\lambda y(x)+f(x)$$ usually can be expressed by means of convolution. We will show that in this way we can get an unbounded LAP solution. We will give an example showing that the convolution in not always a good tool to look for LAP solutions.
Adam Nawrocki
Levitan almost periodic solutions to some linear differential equation
During this lecture we will show that a Levitan almost periodic (briefly: LAP) solution to the equation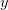 
'3d5clambda20y(x)2bf(x)202424.png)
usually can be expressed by means of convolution. We will show that in this way we can get an unbounded LAP solution. We will give an example showing that the convolution in not always a good tool to look for LAP solutions.